Since we have used different Jovian coordinate systems in previous work (Krupp et al., 1993) we
include a description of these systems. Most of these systems are discussed in Dessler (1983).
The Jovian pole of rotation is defined by the values
![]() given for "Jupiter III" in Table.6.
The transformation from
given for "Jupiter III" in Table.6.
The transformation from ![]() can be calculated from
can be calculated from
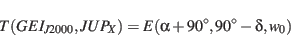 |
(38) |
| +Z-axis: | Pole of rotation. p-angle:
|
| Transform: |
|
This is the 1965 definition of System III, the Pioneer missions used
the 1957 definition:


| +X-axis: | intersection of System III prime meridian and magnetic equator. |
| Transform: |
|
| +Z-axis: | centrifugal axis defined by its System III latitude and longitude: |
|
|
|
| +X-axis: | intersection of System III prime meridian and centrifugal equator. |
| Transform: |
|